contribute of the centrifugal force during deflection
When each weight gets deflected by the small wheel, it accelerates along a radial path (around the pivot point of the one way bearings).
Since the small wheel's linear velocity is higher than the big wheel's velocity,
the centrifugal force that appears at the pivot point of the weight should be
Fcf=m*(delta_v)^2/r
where delta_v is the difference between linear velocity of the small wheel minus linear velocity of the big wheel at a radius where the weights hang from the pivot points. I think this force should not be ingored since it will give a considerable impulse to the pivot point which increases the rotation of the big wheel.
When each weight gets deflected by the small wheel, it accelerates along a radial path (around the pivot point of the one way bearings).
Since the small wheel's linear velocity is higher than the big wheel's velocity,
the centrifugal force that appears at the pivot point of the weight should be
Fcf=m*(delta_v)^2/r
where delta_v is the difference between linear velocity of the small wheel minus linear velocity of the big wheel at a radius where the weights hang from the pivot points. I think this force should not be ingored since it will give a considerable impulse to the pivot point which increases the rotation of the big wheel.






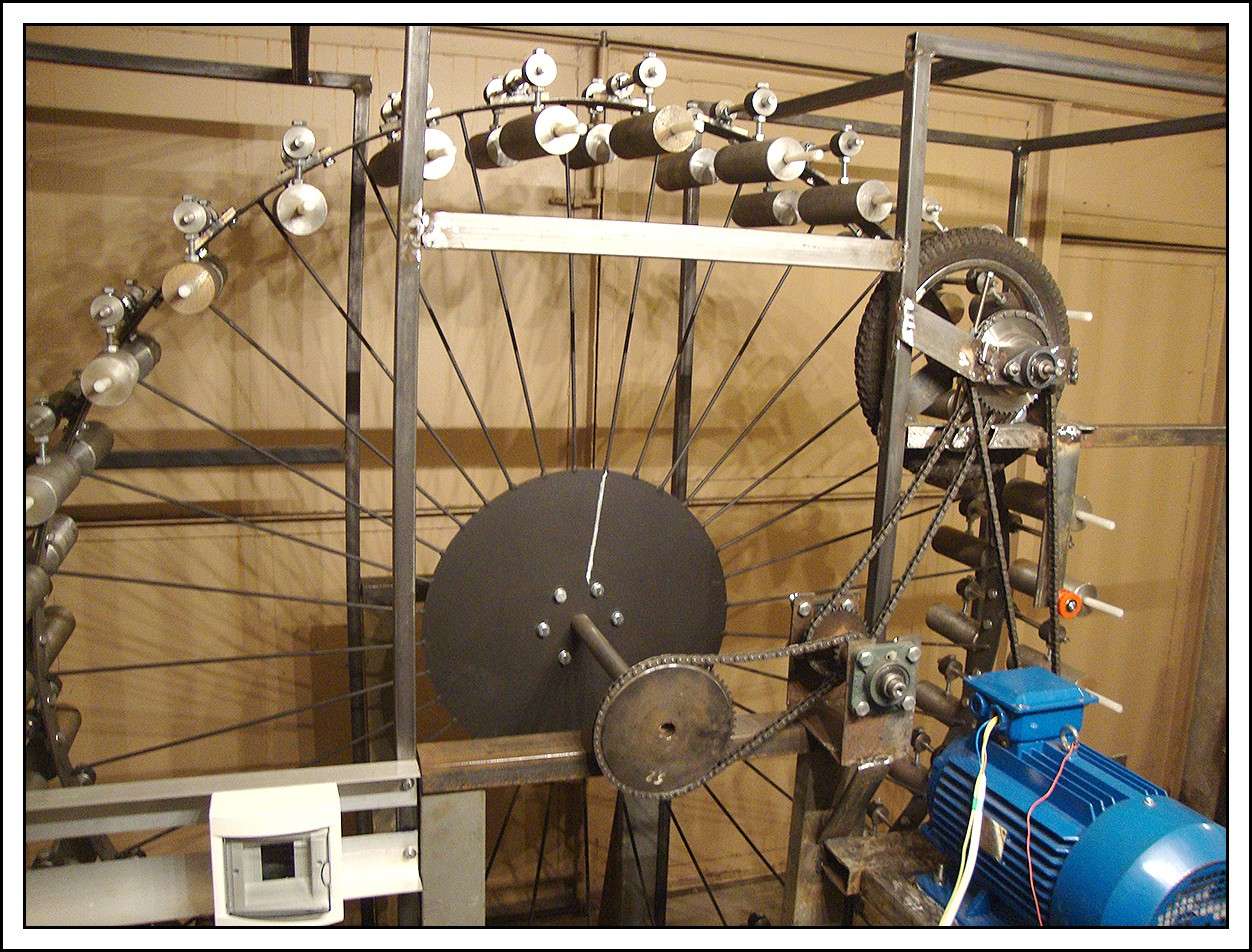
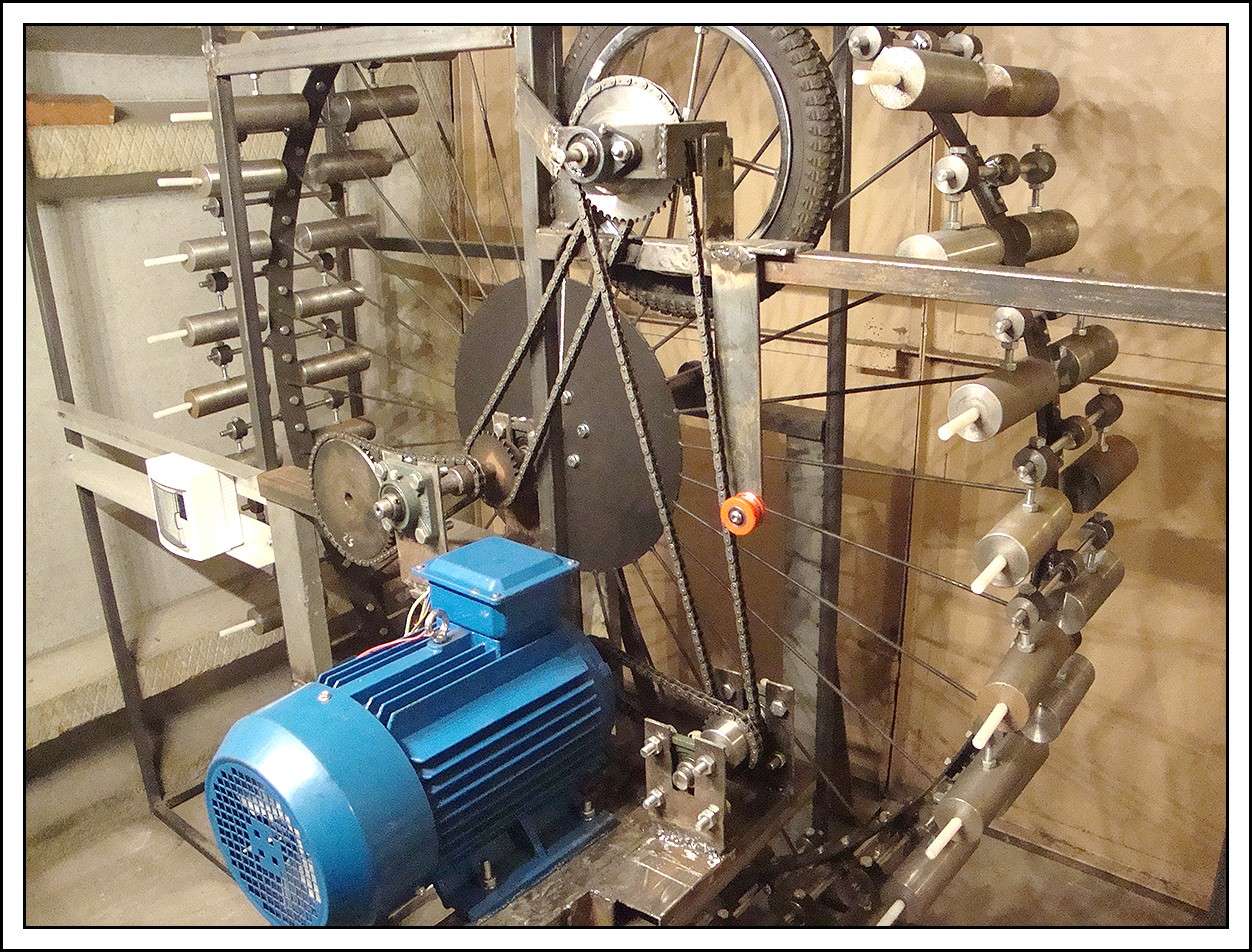
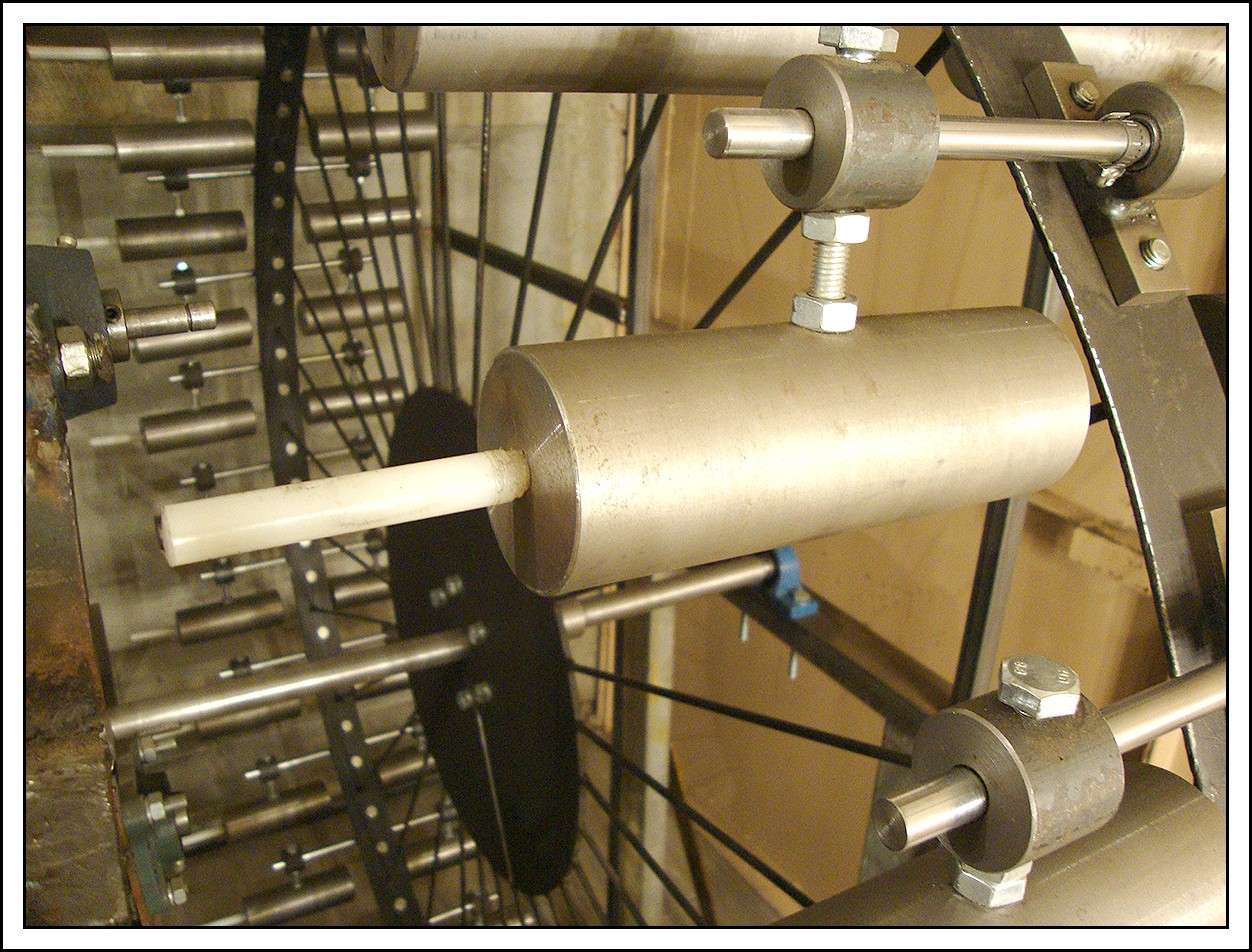
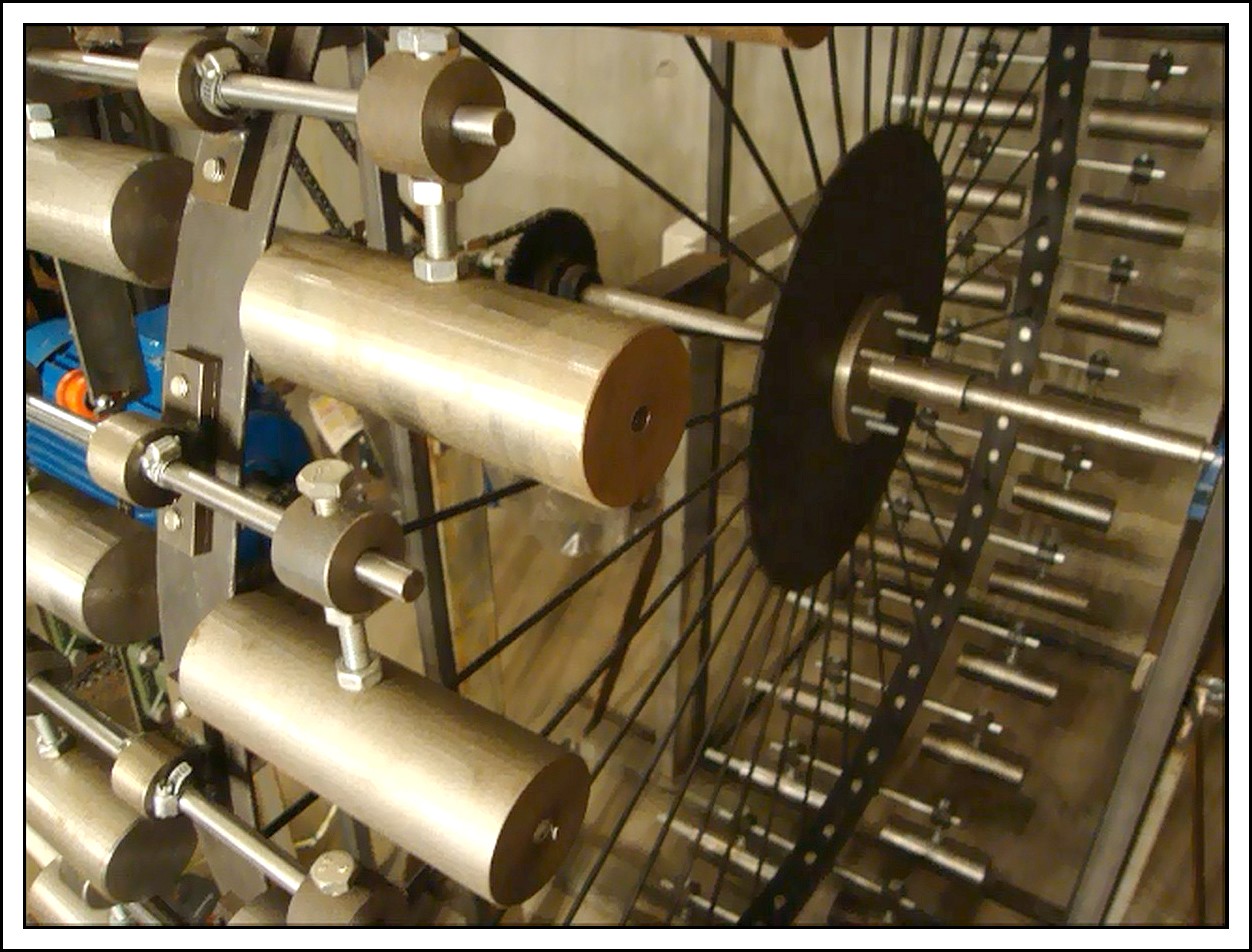




Comment