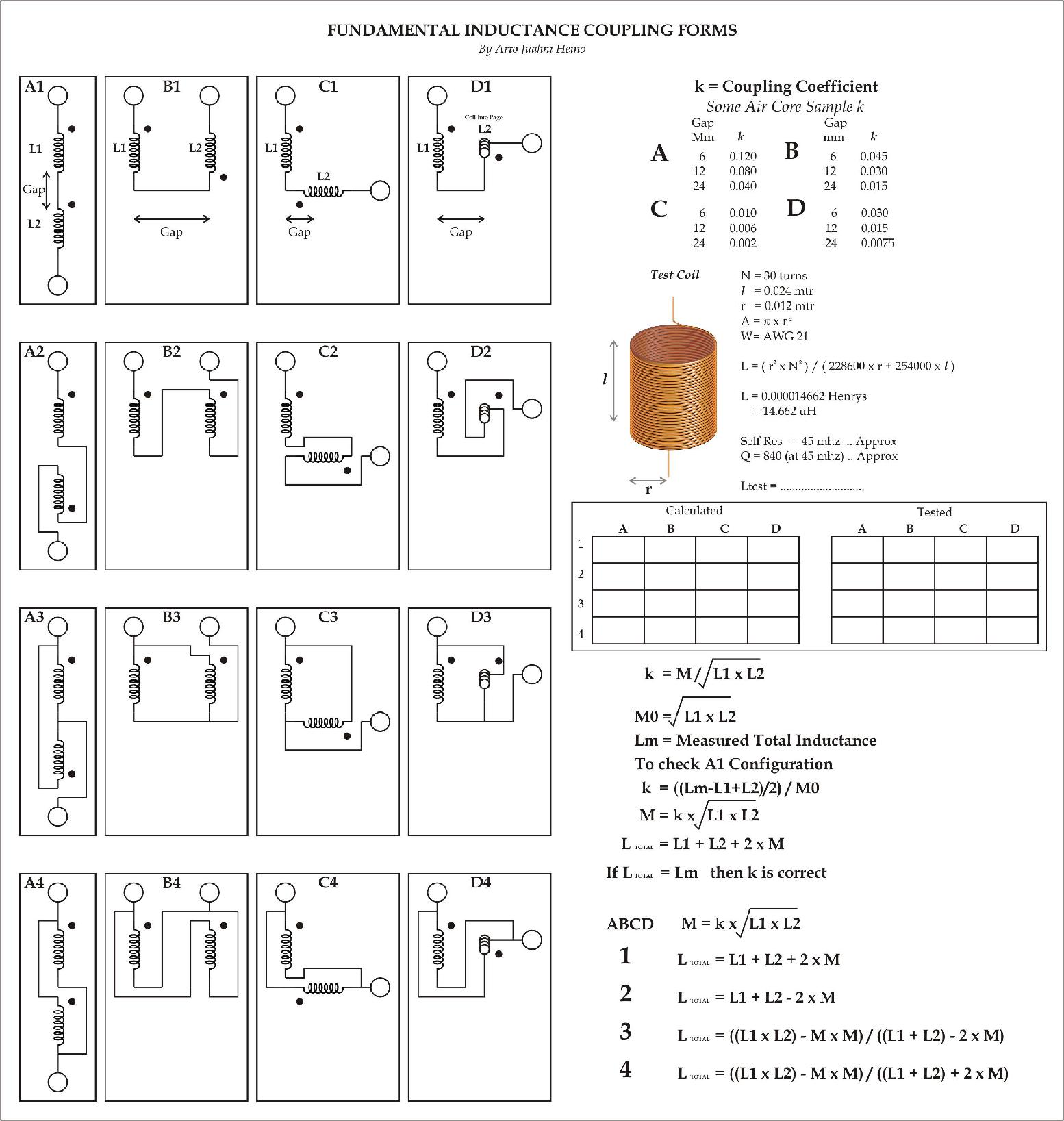
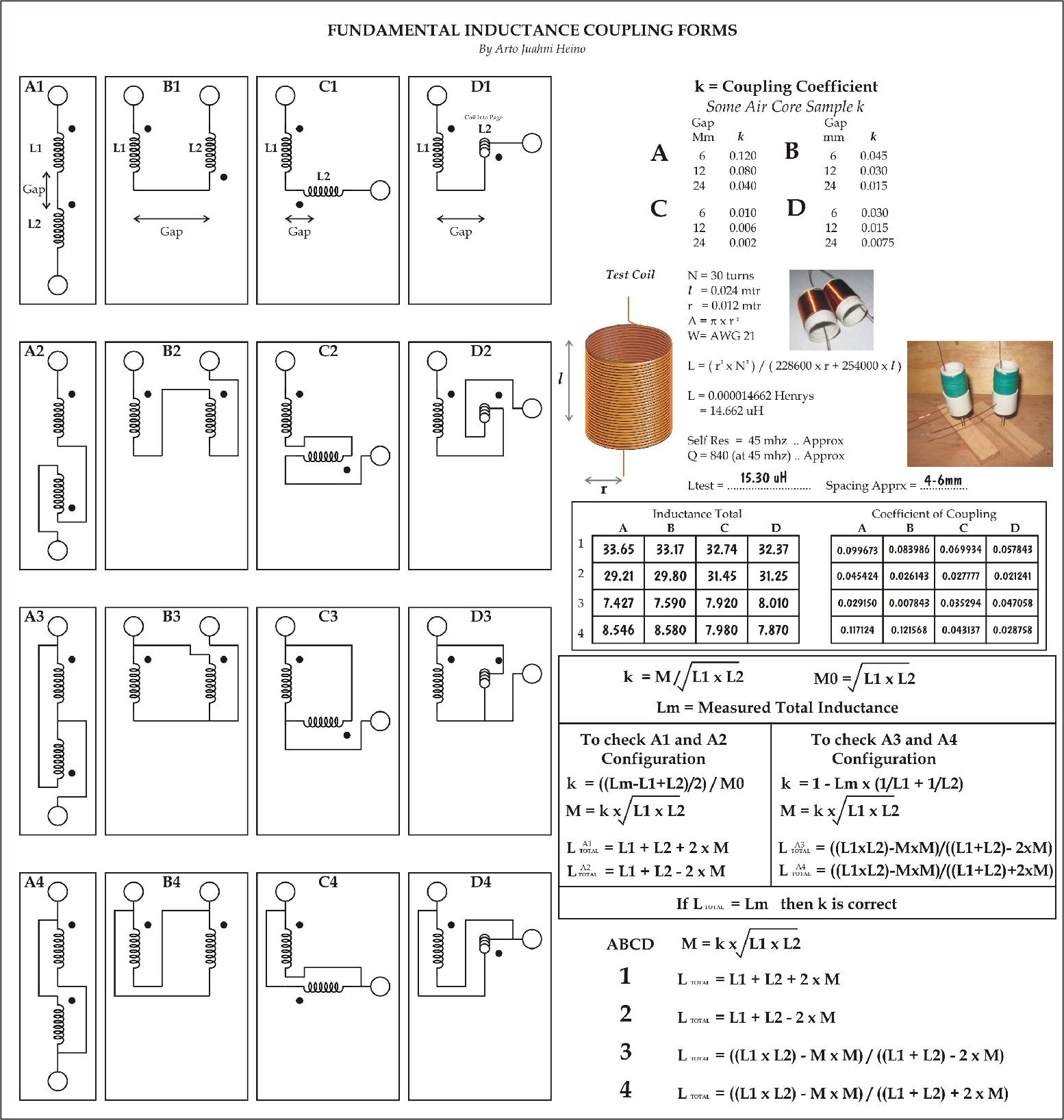
Hi All, I know there are a lot of great engineers and maths people who read this forum, I have a Mutual Inductance chart that I am trying to finish.
I am sure I have missed some simple maths solutions here, please help, I am wasting too much paper scribbling to solve this.
Lt = ((L1xL2)-M^2)/((L1+L2)-Mx2)
I figured this out
Lt = M + 1/(1/(L1-M) + 1/(L2-M))
Then this is far as I have gotten
1/(Lt-M) = 1/(L1-M) + 1/(L2-M)
What I am trying to get a result is
M = *********
If somebody recognizes these type of equations or know
how to solve this, I would very much appreciate some help.
Regards Arto
I am sure I have missed some simple maths solutions here, please help, I am wasting too much paper scribbling to solve this.
Lt = ((L1xL2)-M^2)/((L1+L2)-Mx2)
I figured this out
Lt = M + 1/(1/(L1-M) + 1/(L2-M))
Then this is far as I have gotten
1/(Lt-M) = 1/(L1-M) + 1/(L2-M)
What I am trying to get a result is
M = *********
If somebody recognizes these type of equations or know
how to solve this, I would very much appreciate some help.
Regards Arto


Comment