Just wanted to update here:
I've been researching thru papers and sadly there is little to no advancement in electrostatic equations in non-uniform fields or moving fields. The latest book I have on electromagnetic field theory has a quick toss to electrostatic fields and mostly adds integration and vector plane equations, nothing new other than making it in my opinion a bit more complicated. granted it's a text on electromagnetic fields.
I'm digging for more of JJ Thomsons lectures and not the ones that have been re-printed in latex or text where the diagrams and original formulas have been edited.
From the majority of equations and formulas it is easier to elicit the dielectric circuit and transient waves when starting within or mixing the dielectric and electromagnetic circuits, however it's a compromise and there is a loss of the pure nature of the dielectric field.
for example using some basics, if a charged circuit with zero current and high potential is closed the transition to final current would be instant if there was no inductance and only resistance, however due to the nature of the circuit there is inductance and thus there is a slope for both charge and discharge or rise and fall of the current.
a condenser connected to a constant voltage will have no current, at closing the circuit if there is no resistance or inductance it would have an infinite current and charge the condenser to full potential instantly.
anyone see where this is going?
as the increase in charge on the condenser becomes greater there is less electromotive force for resistance and at full capacitance there is no current.
OK, the trick to this circuit is in near non-resistive materials or super conductors. geometry also plays a big role as well.
If one can generate the field collapse and give rise to an near instant infinite current the current would nearly match the voltage and cycle between charge/discharge with little to no further input.
dielectric study is fascinating as there has been little to no published work in this field for a century yet has such potential and also scary implications at the power achievable.
electromagnetic circuits has great useable current as it's 'regulated' via resistance of inductance, with no inductance the current would be massive and instant. electrostatic circuits are not compatible with electromagnet ones unless a transformation or control in utilized. This is a more noticeable problem within HV transmission and transient waves.
This is also why Steinmetz, Thomson and others have studied transient waves, they arise out of the dielectric circuit that is partially integrated in systems. If the electromagnetic circuit can exclude the dielectric then the dielectric can exclude the electromagnetic.
This is where I'm going. The simple trig and algebraic equations and calculus are falling short. the field is not uniform and I from what I can see in the equations thus far uniform fields will not help in tapping the field. in order to maintain a uniform field the boundary conditions on a condenser need to employ an inductive field and that wastes the energy gain.
assuming a perfectly flat and uniform surface for the field would give rise to an immense attraction and if the surface was lets say gridded to match the full charge density it would collapse uniformly, if it was not and the contact points was less than the charge density it would clamp together will little current flow and be akin two magnets and if the density of any one contact point was greater than the surrounding proportional charge density then the field would break down non-uniformly and there would be a loss in the non inductive current. I don't have the equations to handle that topology or density at the moment.
The equations need to account for many many things and I feel that integration via a uniform field is not correct and will mask or hide the true available nature of the phenomenon.
I've been researching thru papers and sadly there is little to no advancement in electrostatic equations in non-uniform fields or moving fields. The latest book I have on electromagnetic field theory has a quick toss to electrostatic fields and mostly adds integration and vector plane equations, nothing new other than making it in my opinion a bit more complicated. granted it's a text on electromagnetic fields.
I'm digging for more of JJ Thomsons lectures and not the ones that have been re-printed in latex or text where the diagrams and original formulas have been edited.
From the majority of equations and formulas it is easier to elicit the dielectric circuit and transient waves when starting within or mixing the dielectric and electromagnetic circuits, however it's a compromise and there is a loss of the pure nature of the dielectric field.
for example using some basics, if a charged circuit with zero current and high potential is closed the transition to final current would be instant if there was no inductance and only resistance, however due to the nature of the circuit there is inductance and thus there is a slope for both charge and discharge or rise and fall of the current.
a condenser connected to a constant voltage will have no current, at closing the circuit if there is no resistance or inductance it would have an infinite current and charge the condenser to full potential instantly.
anyone see where this is going?
as the increase in charge on the condenser becomes greater there is less electromotive force for resistance and at full capacitance there is no current.
OK, the trick to this circuit is in near non-resistive materials or super conductors. geometry also plays a big role as well.
If one can generate the field collapse and give rise to an near instant infinite current the current would nearly match the voltage and cycle between charge/discharge with little to no further input.
dielectric study is fascinating as there has been little to no published work in this field for a century yet has such potential and also scary implications at the power achievable.
electromagnetic circuits has great useable current as it's 'regulated' via resistance of inductance, with no inductance the current would be massive and instant. electrostatic circuits are not compatible with electromagnet ones unless a transformation or control in utilized. This is a more noticeable problem within HV transmission and transient waves.
This is also why Steinmetz, Thomson and others have studied transient waves, they arise out of the dielectric circuit that is partially integrated in systems. If the electromagnetic circuit can exclude the dielectric then the dielectric can exclude the electromagnetic.
This is where I'm going. The simple trig and algebraic equations and calculus are falling short. the field is not uniform and I from what I can see in the equations thus far uniform fields will not help in tapping the field. in order to maintain a uniform field the boundary conditions on a condenser need to employ an inductive field and that wastes the energy gain.
assuming a perfectly flat and uniform surface for the field would give rise to an immense attraction and if the surface was lets say gridded to match the full charge density it would collapse uniformly, if it was not and the contact points was less than the charge density it would clamp together will little current flow and be akin two magnets and if the density of any one contact point was greater than the surrounding proportional charge density then the field would break down non-uniformly and there would be a loss in the non inductive current. I don't have the equations to handle that topology or density at the moment.
The equations need to account for many many things and I feel that integration via a uniform field is not correct and will mask or hide the true available nature of the phenomenon.
 I have a question for you , because I can't operate the math now (I've got some weird illness which removed all complex or even less complex math from my head) - can you check LC circuit ? I mean I feel that changing magnetic field in coil (both rise and fall slope) should produce displacement current but out common two plates capacitor is wrong to catch it. With proper capacitor geometry it should be possible to accumulate energy in LC circuit expotentially as opposite to current linear way.
I have a question for you , because I can't operate the math now (I've got some weird illness which removed all complex or even less complex math from my head) - can you check LC circuit ? I mean I feel that changing magnetic field in coil (both rise and fall slope) should produce displacement current but out common two plates capacitor is wrong to catch it. With proper capacitor geometry it should be possible to accumulate energy in LC circuit expotentially as opposite to current linear way.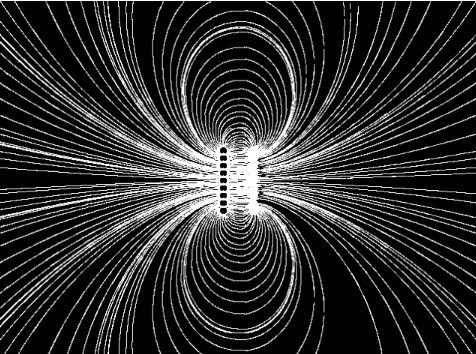


Comment