Announcement
Collapse
No announcement yet.
Eric Dollard
Collapse
This is a sticky topic.
X
X
-
Unless I just flat out missed something obvious about the link that you gave, I still have my same mental block as before. Let me go about conveying my problem by defining my problem. I am interested in being able to correctly plot the permeability (B/H) of a material using a complete hysteresis loop, not one that starts from the origin (0,0), rather the one that creates the full loop.Originally posted by madhatter View PostI thought of trying to explain, but figured it'd take wayyy to much typing, so I'll point you here. Permeability and saturation : Magnetism And Electromagnetism
The horizontal line represents "storage" or hysteresis. hope that helps.

Dotted Line - I understand how to plot the permeability using the formula, mu=B/H.
Solid Line - I am having trouble seeing how to plot the effective permeability using the formula mu=B/H. As H -> 0, mu tends towards (+/-) infinity. Does the permeability really approach infinity? Or is there some mathematical manipulation that can be used the correctly plot the permeability of this BH curve?
I find it hard to believe that the permeability approaches infinity...
Thanks,
Dave
Comment
-
Dave, then the graph starts at 0,0 when there is no applied or stored flux in the core. at the peak or saturation point no further input will increase the cores ability to hold more flux, is that the infinite plane you're referring to? it's not infinite it's that no further increases will cause any change. the stored flux in the core due to the alternating current graphs the solid line from positive to negative axis.
charge and relaxation define that plot. try here: Magnetic properties of materials
Comment
-
-
No, my problem is the instantaneous permeability AFTER saturation occurs, while H is decreasing from a maximum (saturation) back to zero. I am interested only in the equation:Originally posted by madhatter View PostDave, then the graph starts at 0,0 when there is no applied or stored flux in the core. at the peak or saturation point no further input will increase the cores ability to hold more flux, is that the infinite plane you're referring to? it's not infinite it's that no further increases will cause any change. the stored flux in the core due to the alternating current graphs the solid line from positive to negative axis.
charge and relaxation define that plot. try here: Magnetic properties of materials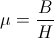 .
.
I am interested in the permeability plot that can be seen here in blue:

In a permeability plot such as the one above (Tendency towards infinity NOT SHOWN in graph due to the fact that it starts at 0,0), the permeability, mu, will approach infinity as H approaches zero after saturation. B is still greater than zero when H becomes zero. When H = 0, permeability is undefined, but as H approaches zero, mu tends towards infinity.
I just don't think that the graphical 'spike' (tending towards infinity) from the plotted mu=B/H is a real expression of the instantaneous permeability of the material under question.
Sorry that I am having trouble conveying my problem..
DaveLast edited by Web000x; 12-20-2012, 02:56 AM.
Comment
-
sorry Dave I don't follow. did you get a chance to go thru the second link?Originally posted by Web000x View PostNo, my problem is the instantaneous permeability AFTER saturation occurs, while H is decreasing from a maximum (saturation) back to zero. I am interested only in the equation: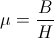 .
.
I am interested in the permeability plot that can be seen here in blue:

In a permeability plot such as the one above (Tendency towards infinity NOT SHOWN in graph due to the fact that it starts at 0,0), the permeability, mu, will approach infinity as H approaches zero after saturation. B is still greater than zero when H becomes zero. When H = 0, permeability is undefined, but as H approaches zero, mu tends towards infinity.
I just don't think that the graphical 'spike' (tending towards infinity) from the plotted mu=B/H is a real expression of the instantaneous permeability of the material under question.
Sorry that I am having trouble conveying my problem..
Dave
Comment
-
Yes, I read the second link, but still didn't find my answer.
Could you describe what the instantaneous permeability is everywhere on the following plot? (Make up your own arbitrary B magnitude and H magnitude scale)

You'll find that when H approaches 0, instantaneous permeability tends towards infinity according to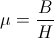 .
.
I have a situation where I would like to know the exact instantaneous permeability for every part of an AC wave in the core. Does the instantaneous permeability tend towards infinity as seen by this simple equation?
Dave
Comment
-
I guess I don't understand the question, I don't see any infinite curvature. the mu of the material starts at zero and is increased with the applied field, the core retains that flux even after the field is removed that is the remnance. are you referring to the point on the Y axis above the zero X?
Comment
-
I want to plot something like the dotted line, permeability, in this graph:
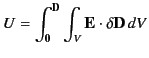
And apply it to this type of graph:
It seems that the B Divided by H relationship of the latter type of permeability plot is suggesting a relatively large B value being divided by a small H value which tends towards infinity as H approaches zero.Originally posted by Web000x View Post
Could you describe what the instantaneous permeability is everywhere on the following plot? (Make up your own arbitrary B magnitude and H magnitude scale)

It may be time to move this discussion off of this thread..
Dave
Comment
-
ahh, that's not directly calculable it's a measured graph. lots of variables and the integration alone would be massive! try this link, Plotting Magnetization CurvesOriginally posted by Web000x View PostI want to plot something like the dotted line, permeability, in this graph:
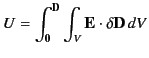
And apply it to this type of graph:
It seems that the B Divided by H relationship of the latter type of permeability plot is suggesting a relatively large B value being divided by a small H value which tends towards infinity as H approaches zero.
It may be time to move this discussion off of this thread..
Dave
Comment
-
Dave, part 2 of the Tesla Ether Tape is in the folder called "Gerry Vassilatos with Laura Lee - Tapes", there are 6 files in there starting with "temp1 2", that is the continuation of that conversation. This is the way I received it, sorry about the confusion, and terribly sorry about the virus, I've never gotten one from that site and that is why I used it, I would never direct someone to a shady site. You'll find that the first file in that folder picks up right where Vassilatos_Tesla_Ether_1 leaves off. I should have combined those files and properly named it first, but at the time I didn't realize that was the continuation of that conversation. Hope it works out for you.Originally posted by David G Dawson View PostLambda,
Tesla Ether Tape #2.
Checked again and is definately missing and all the others appear to be ok.
Tried to download again but won't let me.
There is a Trojan virus attached to the first site which you will need to clean out of your systems.
'Stopzilla' is a good cleaner.
Concen appears to be ok.
Smokey
Mike
Comment
-
Vril Compendium
Mike,
Right, got it!
Many Thanks.
A good chapter on Moray there in 'Lost Science' as well which is most enlightening.
The beauty of reading this material when you believe it comes from a reliable source is that you can begin to develop an organisation of your own that may help to replicate the device.
Have a picture here of a Moray box and all it has inside are 3 x 4" coils and 3 x single gang condensers and there is also another on a flatbed of similar construction, just coils and condensers, no Tubes but lighting a fair sized lamp.
Smokey
Comment
-
mathematical Problem
Originally posted by Web000x View PostNo, my problem is the instantaneous permeability AFTER saturation occurs, while H is decreasing from a maximum (saturation) back to zero. I am interested only in the equation: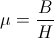 .
.
I am interested in the permeability plot that can be seen here in blue:

In a permeability plot such as the one above (Tendency towards infinity NOT SHOWN in graph due to the fact that it starts at 0,0), the permeability, mu, will approach infinity as H approaches zero after saturation. B is still greater than zero when H becomes zero. When H = 0, permeability is undefined, but as H approaches zero, mu tends towards infinity.
I just don't think that the graphical 'spike' (tending towards infinity) from the plotted mu=B/H is a real expression of the instantaneous permeability of the material under question.
Sorry that I am having trouble conveying my problem..
Dave
Hi Dave,
as we do not consequently, correctly express the functions B(H) and B'(H) = µ(H), we have a little problem at point H = 0. Thats all.
It is not allowed to divide by Zero! So we must consider the lim-function for µ(H) at point H = 0. We have: lim +>0 = lim ->0, so the [Gradient of B(H)] = µ is defined and steady.
Number is: ... put a ruler at the function B(H) at point H = 0, and measure the steepnes delta B / delta H.
Best regards
magnetO
E.P.D. has never answered to my proposal?
No, problem! Must I do by myself.
Comment
-
It is all very well showing these simplistic macro hysteresis plots etc., but they do not hold when relative core, domain or magnet movements occur independently of but simulataneously to coil energisation/ transduction, as may be witnessed with Barhkausen Effect field (spin wave) avalanches.
Comment
-
Thanks madhatter,Originally posted by madhatter View Postahh, that's not directly calculable it's a measured graph. lots of variables and the integration alone would be massive! try this link, Plotting Magnetization Curves
The picture at the end of that article describes exactly what I was looking for. I had a sneaking suspicion that that was the case but couldn't find a good clear cut answer until now.
Dave
Comment
Comment