I'm trying to understand the secondary coil design. It sounds like Nhopa is saying that Tesla's colorado springs notes describe a long thin secondary, yet Eric's notes in intro to tesla coils describe the secondary as a spiral or short fat coil. Anyone want to weigh in on which is correct? The picture on the front of Eric's intro to tesla coils looks like a long thin coil, so I'm confused on what is correct.
Announcement
Collapse
No announcement yet.
Peter, whatever happened with Eric P. Dollard?
Collapse
This topic is closed.
X
This is a sticky topic.
X
X
-
The Colorado Springs coil (the one in most pictures) secondary is 15 metres diameter and about 2m high. Or short and fat.Originally posted by skaght View PostI'm trying to understand the secondary coil design. It sounds like Nhopa is saying that Tesla's colorado springs notes describe a long thin secondary, yet Eric's notes in intro to tesla coils describe the secondary as a spiral or short fat coil. Anyone want to weigh in on which is correct? The picture on the front of Eric's intro to tesla coils looks like a long thin coil, so I'm totally confused on what is correct.
A flat spiral coil is another coil altogether. In context it means you can either build one or the other.
May I recommend going to the source of the information rather than interpreting interpretations
[edit] I suppose the simple answer to your original question if you don't want to have to make an extra coil is to make a flat spiral coil instead.Last edited by dR-Green; 01-28-2012, 03:54 AM.http://www.teslascientific.com/
"Knowledge is cosmic. It does not evolve or unfold in man. Man unfolds to an awareness of it. He gradually discovers it." - Walter Russell
"Once men died for Truth, but now Truth dies at the hands of men." - Manly P. Hall
Comment
-
Hallelujah!
WOW!Originally posted by T-rex View PostFour Quadrant Energy Exchange in Magnetic & Dielectric Fields of Induction, Part One:
That mega post by Mr. Dollard answered quite a few complex and downright perplexing issues that I was trying to resolve when doing the math on impulse discharges (of L or C or both one into the other)! Also the concept of the battery as having characteristics of both a capacitor and inductor rings true with me, I have been trying to resolve how to analyze the storage battery (in the electrical sense, or non-chemical sense) for quite some time and came to similar but not quite as refined results. I think one word would sum up my opinion of what was recently posted, Hallelujah! I feel as if that post filled in a fair bit of what I was unsure about in my post "Question On Plank, Q with Respect to an Impulse Discharge of L or C into r".
Furthermore, we now have new insight into the whole negative resistance / negative conductance situation, I personally HATE the term NEGATIVE RESISTANCE -r (generally this is actually a non-linear/non-ohmic resistance and has nothing to do with excess energy), negative ohms is acceptable by my standards though. With whats presented by Mr. Dollard we at least can now call negative ohms & siemens, Receptance H & Acceptance S, something that makes me very happy indeed. This should elucidate, if the concept is fully understood and used properly, interesting phenomena in an electrical circuit whereby there is an excess of energy (or the "load" acts as a "source").
Finally we see some use of a versor operator and its application and (inferred) theory of its use mathematically in electrical situations! I think there's a lot yet to be said or done with this mathematical tool. Hopefully we will get another post with its usage soon from Mr. Dollard.
In conclusion I feel everyone should read that post a few times over, it has some gold nuggets that are sure to give new insight and potential use in future "practical" real life situations.
Garrett MLast edited by garrettm4; 01-29-2012, 11:33 PM.
Comment
-
Thank you dR-Green for the help with my questions. I guess no.:2, the "well as a ground" still needs to be resolved. It seems to me from the foregoing discussions, that the best answers to questions is experimenting. After all Tesla's Colorado Springs Notes are about experimenting. Tesla did'n have all the answers but deducted many from the various experiments. I also apologize for my mistake about the diameter to lenght ratios of the secondery coil. Most of the "extra" coils have a D to L ratio of larger than 1. For example from the Notes on p.110: 2' dia to 6' lg, p.160: 6' dia to 12' lg. However the cage type "extra" coil shown on a few pages in the Notes are indeed close to the 1:1 ratio.
I agree for first hand information one must be going to the source. In our case the real McCoys are the Colorado Spring Notes and Eric Dollard's publications on the subject.
@skaght: Things can be confusing and that is the reason why I have lots of questions. I would recommend obtaining a copy of the Notes as an onhand reference together with Eric's various publications on the subject.
Comment
-
"image coil"
In Eric's notes, intro to tesla coils, he states that for plasma work you need an "image coil" between the secondary and ground to provide 180 degree phase shift. I would prefer not to build two tesla coils as he seems to suggest later on for plasma work, since I'm not positive of all the design parameters. Would a simple coil with 1/2 wavelength resonance work as an image coil? Or does "image coil" literally mean that the coil is a "mirror image" of the secondary and extra coil? I've been searching for a definition of an image coil and I'm not finding much.
Does anyone know the definition of the term "image coil"?
Thanks!
Comment
-
Hi,Originally posted by skaght View PostIn Eric's notes, intro to tesla coils, he states that for plasma work you need an "image coil" between the secondary and ground to provide 180 degree phase shift. I would prefer not to build two tesla coils as he seems to suggest later on for plasma work, since I'm not positive of all the design parameters. Would a simple coil with 1/2 wavelength resonance work as an image coil? Or does "image coil" literally mean that the coil is a "mirror image" of the secondary and extra coil? I've been searching for a definition of an image coil and I'm not finding much.
Does anyone know the definition of the term "image coil"?
Thanks!
He mentioned AFC - automatic frequency and phase control.Probably need to keep resonance all the time or need to check phase between 2 coils, even load changed. to do that he have 2 same secondary coils. He is measuring phase of 2 secondary coils and based on that is controlling a voltage controled oscillator - VCO.VCO is generating a main fequency and as well a synchronization pulses for pulse modulation of main frequency.Phase discriminator is checking a difference in phase and frequency of 2 signals.It is used in nearly all RF receivers.
On Page 13 of that book is :
"To confine the energy an image coil
(180 degree shift) must be connected to the earth terminal. Making this
arrangement in a horseshoe configuration produces intense dielectric
flux and displacement current that is quite usefull for plasma work."
The electrical length is 360 degrees at the fundamental of oscillation.
I think that image coil is to keep total electrical lenght in 360 degree. then need to control a phase of that 2 coils to get strongest effect.
Comment
-
Versor Operators Decoded, With Respect to DC & AC Circuits Pt1
Foreword:
I thought I would share what I understand about the DC & AC versor operators of Mr. Dollard and while I can’t speak for Mr. Dollard or say that I am 100% right, this post is intended to assist anyone who would like a “beginner’s guide” on the subject (sadly this is all I am capable of at the moment). I feel that Mr. Dollard takes for granted, his understanding of exponentiation and use of versors in relation to degrees of rotation, much like breathing or walking most people don’t even have to think to do these things. Thus this is written for your “common man”, who like myself (I’m a high school dropout), were never given a good education and lack the fundamentals of more advanced math principles. This is by no means a formal study, but instead, can be looked at as a primer on the subject, at least until a better treatment by Mr. Dollard is available.
If you already have a good grasp of the Dollard type versos then this whole post can be skipped over.
Roots & Powers; Exponentiation Cliff Notes:
To fully understand the machinery of a versor operator of the type used by Mr. Dollard, one must first understand the subject of “exponentiation” (also known less formally as roots and powers of a number) or at least have available a basic reference sheet of what each degree of power or root does.
So as not to be confused with the versor operators used latter on, we will use the letter b as our variable and n as our root or power. This exercise requires only basic high school algebra, and will be found to give good insight into the workings of a versor operator.

Here we have b, or some “arbitrary number”, to the nth power, n being another “arbitrary number”. Where b & n are assumed to be whole real numbers and where b is not equal to zero.
Depending upon what “arbitrary number” n represents, changes a whole host of things with how b is treated. Shown below are some simple common numbers that n could represent, and from understanding these seven different powers of b we can start to gain some insight into versors and also what to do when confronted by these in other mathematical situations.

Here we have a few arbitrary values of n, some of which may seem familiar and others very foreign.
Let’s start off with the case of b^1

Here it is understood algebraically that any number raised to the exponent 1 is simply the same number. (In the case of Mr. Dollard’s DC Versor, h=-1 and AC versor k=+j)
Next, we have the case of b^0

Here it is understood algebraically that any nonzero number raised to the exponent 0 will ALWAYS equal POSITIVE UNITY +1. As was shown above for b^1, and referenced with b^0, any number (aside from zero) be it negative or positive divided into itself will always be a positive one.
Moving on to b^(1/2) and b^(1/n)

Here it is understood algebraically that any number raised to the exponent (1/n) represents the root of that number, where n=2 is the “square root”, n=3 the “cube root”, n=4 the forth root etc. This relation b^(1/n) has use with both the DC & AC versor operators, h & k, which will be discussed further on.
Continuing to b^-1

Here it is understood algebraically that any number raised to the exponent -1 is simply the reciprocal of the number itself.
Taking this concept further b^-(1/2) and b^-(1/n)

Here it is understood algebraically that any number raised to the exponent –(1/n) is simply the reciprocal of the root of that number.
It can be seen that these less common “powers” of b can save quite a bit of space when writing an equation and are generally used in calculus for this very reason. Also it can be seen that when using “fractional” powers (1/n) instead of roots (numbers inside a radical), you gain a greater understanding of what is going on mathematically and are less likely to be confused when in unfamiliar circumstances, i.e. fractional powers are roots and negative powers are reciprocals. The cases of b^n where n>2 or b^-n where b<-2 and where b is not equal 0, are very simple cases, which I don’t think need even the slightest explanation, as they are the most commonly understood form of powers (at least for positive powers equal to or greater than 2 this is the case). This topic could be taken much further but I don’t feel there is anything to gain with respect to understanding of the versor operators used by Mr. Dollard in doing so. The most vaguely understood cases of n have been shown and given proper, albeit very simple, explanations with some minor references to the DC & AC versor operators h & k for use further on in this post.
The Roots of Unity; Negative & Imaginary Numbers Cliff Notes:
It turns out the subject “roots of unity” has a lot to do with a Dollard type versor operator and a whole host of other branches of science. With what we just covered on exponentiation we can now briefly cover this primer before finally getting to the versors.

In a nut shell, any number that equals 1 when raised to some power of n is a so called “root of unity”.
When we go to solve for b we get

Here things start to get interesting with regards to Mr. Dollard’s work and his versors.
For the purpose of this post, we will limit the values of n, in the above, to only 2 & 4, as these have the most relevance to the Dollard DC & AC versors.
In the case of n=2 we have

Here we are confronted with the “square root” of unity. From this problem, we get two possible solutions or roots, b=1 & b=-1.
As we all blink our eyes and breathe without giving a second thought, we also take for granted the concept of negative numbers. If we represent positive numbers extending to the right of zero, we can represent negative numbers extending to the left all of which is along a single axis where the angle of measure between both sides is always 180°, as shown below.

The above expression and diagram give some general insight into the DC versor h. The base value of h in the case of Mr. Dollard’s DC versor is always -1, h^1=h=-1. In this special case h^-1, h, h^1, … etc. (all odd non-fractional powers of h^n where n=1+x2) equal -1, or 180° rotation (from +1) and for h^-2, h^0, h^2, … etc. (all even non-fractional powers of h^n where n=0+x2) equal +1 or 0° rotation (from +1).
In the case of n=4 we have

Here we are confronted with the “fourth root” of unity. From this problem, we get four possible solutions or roots, b=+1, b=-1, b=+j=(sqrt-1) & b=-j=-(sqrt-1).
It turns out that a mathematician named Gauss extended the concept of the real axis by placing j or (sqrt-1) as halfway between +1 and -1, having an angle of 90° from the line -1 and +1. If the division of unity into plus and minus is 180°, a second division leads to an axis which intersects this line at an angle of 90°. We now have two axes, the horizontal representing positive and negative real numbers, and the vertical representing positive and negative imaginary numbers. These two axes form the complex coordinate system, any number inside this plane, is a number having a real and an imaginary part.

The above expression and diagram give some general insight into the AC versor k. The base value of k in the case of Mr. Dollards AC versor is always +j, k^1=k=+j, also the diagram above would need to be turned 90° to the left so that the positive real axis is on top. In this special case, k, k^1, k^5, … etc. (all odd non-fractional powers of k^n where n= 1+x4) equal +j, or 90°, rotation (from +1), for k^0, k^4, k^8 … etc. (all even non-fractional powers of k^n where n= 0+x4) equal +1 or 0° rotation (from +1), for k^2, k^6, k^10… etc. (all even non-fractional powers of k^n where n= 2+x4) equal -1 or 180° rotation (from +1), for k^3, k^7, k^11… etc. (all odd non-fractional powers of k^n where n= 3+x4) equal -j or 90° rotation (from +1). Note that negative values for exponents are not shown, but would follow the same pattern, if I’m not mistaken, as for the positive values as they are only reciprocals, i.e. 1/j=j & 1/-1=-1.
Continued in Part 2Last edited by garrettm4; 02-02-2012, 10:12 PM.
Comment
-
Versor Operators Decoded, With Respect to DC & AC Circuits Pt2
Continuing with Part 2
Versor Operators; Rotation about an Axis:
With the above cliff notes on exponentiation and roots of unity we can now perform a more advanced and elucidated study of a Dollard type versor operator, but first, we should cover a few basic concepts of what a versor is and historically where it came from.
For this purpose, one needs go no further than a quick study of William Rowan Hamilton, the discoverer of quaternions. Hamilton during the year 1843, discovered a new mathematical entity which he called a quaternion and from which formed a new branch of mathematical thought that extended the complex numbers, with new imaginary axes “j” & “k” (“i” is the familiar imaginary axis from high school). This expanded the usual Argand diagram with two new axes that have a 90° separation to the Re and Im axes while also having a 90° separation between themselves, j & k. New terms were spawned from the mind of Hamilton to describe the unique concepts and entities contained in this seemingly odd branch of mathematics. Notably the most important, for our discussion, are “scalar”, “tensor” “vector” & “versor”. Without going too deep into this topic, we will only cover the versor and a few of its associated relations.
Hamilton’s Versor
In Hamilton’s branch of math, each Quaternion has a Tensor, which is a measure of its magnitude; this being similar to the length of a Vector is a measure of a Vectors' magnitude. The Versor is a Quaternion having a Tensor of 1, Tensors by Hamilton’s definition are “signless numbers” or more simply a positive number. The term Versor was derived by Hamilton from the Latin vertere, "to turn".
In general a Versor can be associated with a plane, an axis and an angle. When a versor and a vector which lies in the plane of the versor are multiplied, the result is a new vector of the same length but turned by the angle of the versor. When the arc of a versor has the magnitude of a right angle (π/2), it is then called a Right Versor. Right Versors have a zero Scalar part, and thus, are Vectors of length one, or Unit Vectors.
Outside the context of quaternion theory, in normal algebra and geometry, the term Versor is often used for a Right Versor. In this case, a Versor is defined as a Unit Vector indicating the orientation of a directed axis in a Cartesian coordinate system.
Dollard type Versors
It can be seen that the Dollard DC & AC versors share many commonalties with Hamilton’s Quaternion Versor, but despite this, they generally have little to no relation to one another. The Dollard versors, from what I understand, are used to indicate the orientation of (or rotate) a vector magnitude’s position in a Cartesian coordinate system, and possibly polar or spherical coordinate systems as well. These versors form what could be called a Unit Vector, where the vector magnitude is always equal to unity.
In the case of both the DC & AC versors, their associated Unit Vector always equals a magnitude of one, also, the possible “directions” of travel are associated with the number of axes present. This is where we get the accompanying roots of the Unit Vector. The number of possible directions become the exponent n of the unit vector and the corresponding roots derived, are from the number of potential positions, these derived roots represent the angle of rotation.
The DC Versor h, 180° Rotation (or Mirror Image Operator)
Thus, if there are only two possible directions on the real axis, as seen from the origin (+1 & -1), such as in the DC circuit (consumption and production of active power) we have:

With the real axis as the only number system used, we have only two directions of travel, thus the versor operator h has only two possible positions with corresponding values +1 & -1. Despite any arbitrary power of h (-1), there is a periodicity or repeating nature of the values for h, which can be seen as a cycling through or rotation of the positions that h can have. For simplicity, only h^1 (or h) & h^0 are needed to describe -1, 180° rotation (from +1) & +1, 0° rotation (from +1). The pattern of periodicity for h was discussed earlier under the topic “roots of unity”.
The AC Versor k, 90° Rotation (or Quadrant Operator)
Note: When dealing with Mr Dollard's AC versor k, the usual Argand diagram would need to be turned 90° to the left so that the positive real axis is on top. Everything I have written in this post, related to versor k, is in direct reference to this subtle change of perspective! i.e. the horizontal or "x" axis is now the imaginary axis and the corresponding vertical or "y" axis is now the real axis.
Similarly, if there are four possible directions along the real & imaginary axes, as seen from the origin (+1, -1, +j & -j), such as in the AC circuit (production of active & reactive power and consumption of active & reactive power) we have:

With the real & imaginary axes, as the number systems used, we have four directions of travel, thus the versor operator k has four possible positions with corresponding values +1, -1, +j & -j. Despite any arbitrary power of k (+j), there is a periodicity or repeating nature of the values for k, which can be seen as a cycling through or rotation of the positions that k can have. For simplicity, only k^1, k^0, k^2 & k^3 are needed to describe +j, 90° rotation (from +1), +1, 0° rotation (from +1), -1, 180° rotation (from +1) and –j, 90° rotation (from +1). The pattern of periodicity for k was discussed earlier under the topic “roots of unity”.
Something of great interest to this concept, is the fact that if an arbitrary vector is multiplied by k^1, the rotation is always equal to one -90° (or +270°) rotation (relative to where it was), where a vector of (j,1) is multiplied by k^1, it would be rotated -90°, or now positioned at (j,-1). When a vector is multiplied by k^0, the rotation always equals 0°, or no rotation (even if it were 360°, there was no apparent change of position), where a vector of (j,1) is multiplied by k^0, it would still be positioned at (j,1). When a vector is multiplied by k^2, the rotation is always equal to one +180° (or -180°) rotation (relative to where it was), where a vector of (j,1) is multiplied by k^2, it would now be positioned at (-j,-1). When a vector is multiplied by k^3, the rotation is always equal to one -270° (or +90°) rotation (relative to where it was), where a vector of (j,1) is multiplied by k^3, it would now be positioned at (-j,1).
Note: The coordinates used for the above arbitrary vectors, is in the standard format where (x,y) denotes the exact position on the grid, and where the length or MAGNITUDE of the vector is determined as the distance from the origin (0,0) to that point.
The notion of whether the RESULTANT rotation, by k^n, is NEGATIVE (-) or POSITIVE (+), has strictly to do with the "NORMAL" direction of rotation of the arbitrary vector. In the special case of vectors E & I, the INDUCTIONS of the Magnetic & Dielectric fields, we find that they counter rotate, and thus form a pair of counter rotating vectors, having a double frequency PRODUCT (EI=P) called Power. E is said to rotate Clock-Wise (CW) and I is said to rotate Counter-Clock-Wise (CCW) with this distinction made, a rotation by versor k can now be found to have produced a forward (leading) or backward (lagging) effect on the vector's arbitrary position, with respect to its normal direction of rotation.
I hope this helps anyone who was a bit unsure as to what Mr. Dollard’s versors were all about, granted there is quite a lot of regurgitation of various other concepts I feel that they were necessary to cover before a good grasp of the versor (as a unit vector) concept could be had.
Continued in Part 3, The subtopic of "AC Power" is discussed in minor detail, which shows the relevant "cause & effect" brought about by a rotation by versor k.
Garrett MLast edited by garrettm4; 02-03-2012, 02:53 AM.
Comment
-
Could this be what tesla had in mind?
the earth as a spherical capacitor inside the air as an outer spherical capacitor with the coils connecting the whole thing as a gigantic resonant tank?

and does anyone have an opinion on what naudin did here?

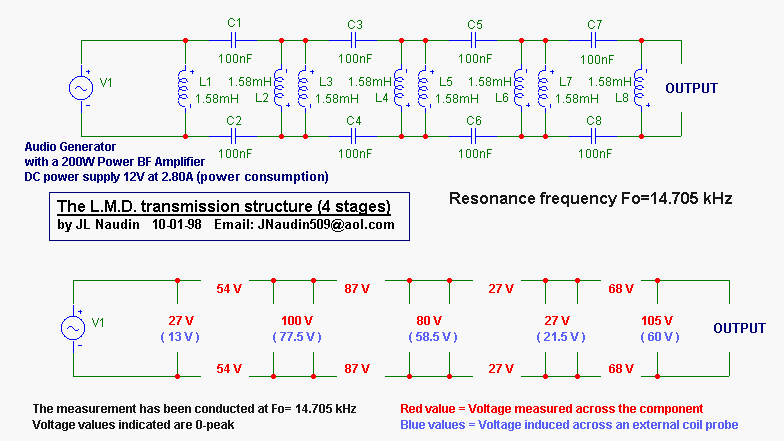
 You may notice that the Magnetic energy is in Space CONJUCTION with the Dielectric energy
You may notice that the Magnetic energy is in Space CONJUCTION with the Dielectric energy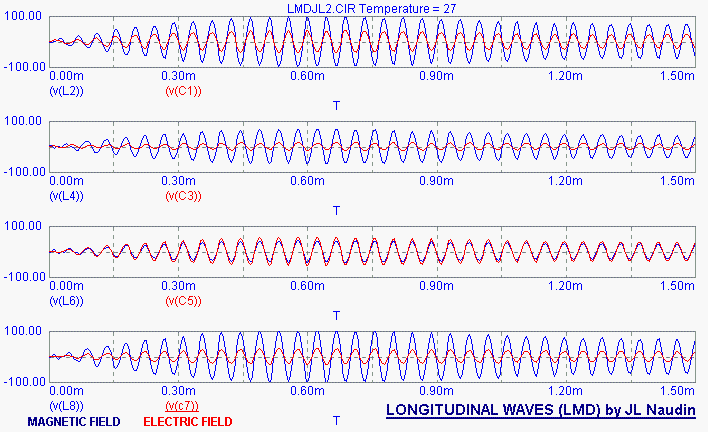



appears he replicated Erics setup in part.
see the rest here
Has anyone talked with him about this and how he made those conclusions?
I know Eric got higher voltage, but according to naudin as shown above greater power out as well?
Find that a bit difficult to accept.
Last edited by Kokomoj0; 02-01-2012, 03:46 AM.
Comment
-
Thanks Garretm4 / LMD setup
@garretm4:
For some simple feedback on your extensive efforts on Vensor Operator decoding of AC & DC circuits, yes I have found it useful in helping to better understand some of Mr Dollard’s maths. Unfortunately I do not quite grasp all of the advanced maths principles, so yes, it does help. Thank you.
@KokomojO
As for the LMD experiment tests first done by Mr Dollard, and reproduced by Naudin: I am unsure about the power output shown by Naudin.
Having said that, I would suggest you just give it a go yourself, even just for experiment sake!
I have played around with these of recent times and also going back a few years ago too. My setup at the moment consists of 300watt audio amp, fed by an audio generator. Two inductors of 4mH and of about 7ohms each. Wound on 3.5 inch PVC pipe in the conventional way with 0.5mm wire. – (Eric would not approve). Two caps of 0.005uF at 10,000 Volts, oil filled, (oil caps seem to work the best but are $$). (I will soon complete two more coils and two more caps). This setup resonates at around 50kcps. Basically, I think it ends up as being (two) single wire transmissions. Each output will easily light two large fluro tubes. If they are kept open circuit the fluro’s radiate well. Bringing another fluro, (just holding it) near, it also lights up.
Using a single fluro’s on each end (output) one can feel tingles through the glass of the fluro tube. If a single fluro tube is put right across the output (closed circuit) one can feel a charge coming off of the fluro and if I’m not dreaming one can also feel a delicate pressure given off too. (?) It feels “nice”. Rectifying the output to DC and then charging caps is another area of interest. (I’ll say no more at this time).
Basically many of the effects from normally seen with a Tesla coil can be seen with this setup. - Mostly when the system runs in open circuit mode. The amplifier sees a resonant series circuit as a short circuit, so the ohms of resistance of the coils is the only impedance the amp works with, so the ohms are about 3.5ohms, but the amp transistors still get hot! As for power in and out, I have not measured as I don’t have high frequency meters at the moment so I have not measured. Voltage is high! However I have only just observed the “effects” only. - Give it ago yourself."Doesn't matter how many times you kick the coyote in the head, it's still gonna eat chickens". - EPD
Comment
-
Versor Operators Decoded, With Respect to DC & AC Circuits Pt3
Continuing with Part 3
Note: While this portion of my post is not paramount for the understanding of the AC versor k, I do feel the subject of AC Power is quite mysterious for most people and thought I would attempt to shed some light on the matter.
AC Power; Complex, Absolute, Real & Imaginary:
Whether the current I or voltage E of the AC circuit is leading or lagging, by any arbitrary value (in degrees or radians), the resultant QUADRANT in which the vector of Power is located changes as a direct result. There exist four quadrants in the Cartesian coordinate system, which form the complex plane as seen on an Argand diagram, each quadrant can be thought to represent a specific condition or state of the electric circuit. These specific states are CONSTANT for ANY circuit whose PARAMETERS (L, C, M, K, g & r) DO NOT CHANGE in TIME. If the load of an AC motor increases or decreases a change in the MAGNETUDE of transferred magnetic energy from L1 to L2 via M causes a STATE CHANGE, thus a corresponding change in the POSITION of the Power vector. For the very SPECIAL condition of of a storage parameter (L or C) whose value changes PERIODICALLY in time, we will find that the Power vector will have two or more PERIODIC positions in TIME. Determined by the state of affairs present, this special condition has the possibility to REMOVE energy from the circuit (with out associated loss as heat) while also having the possibility of INTRODUCING energy into the circuit (from the fields of induction OUTSIDE the guiding wires).
As is shown in Mr. Dollards (excellent) book Symbolic Representation of Alternating Electric Waves (Pages 28-29)
The Real & Imaginary, two Axes having Four Directions:
(+1) 0, Consumption of Active Energy
(+j) 1, Consumption of Reactive Energy
(-1) 2, Production of Active Energy
(-j) 3, Production of Reactive Energy
If the vector of Power is found to be on one of the axes, it is seen to have a 100% condition of any one state, dependent upon its direction. i.e. 100% consumption of active energy or 100% production of active energy.
If the vector of Power is found to lie outside the vertical and horizontal axes, such as between axes (which is the usual case), it will be found inside only one of four quadrants.
The Corresponding Quadrants:
(+j,+1) I Consumption of Active & Consumption of Reactive Energy
(+j,-1) II Production of Active & Consumption of Reactive Energy
(-j,-1) III Production of Active & Production of Reactive Energy
(-j,+1) IV Consumption of Active & Production of Reactive Energy
The exact placement of the Power vector inside any one quadrant will yield any arbitrary GRADIENT of the above listed states. This causes the complex problems associated with MEASURING the MAGNITUDE of the Power vector. This is because we have EIGHT possible conditions in which the state of "Power" can be in (four single states, on an axis & four dual states, between axes), thus no single measurement will suffice in giving the total measurement of Power.
Hence the four Measurements of AC Power:
1 Apparent Power..(Watt Meter + VAR Meter)..(Complex, Real + Imaginary Axes Value)
2 Vector Power.....(Volt Meter * Amp Meter)...(Absolute, sqrt(Real^2 + Imaginary^2) Axes Value)
3 Active Power.....(Watt Meter)....................(Real Axis Value)
4 Reactive Power..(VAR Meter).....................(Imaginary Axis Value)
The length or MAGNITUDE of the Power vector is determined by the distance from the origin (0,0) to its location (x,y), this length is what we "MEASURE" when using our watt, var, volt & amp meters. In the case of Apparent Power we add the length of the active power (0,0) to (0,y) to that of the reactive power (0,0) to (x,0), this forms a complex number which cannot be found with only one measurement. This geometrically analyzed, would be seen as a corner of a square or rectangle. For the measurement of Vector Power we take the actual length of the Power vector, or its distance from (0,0) to (x,y). Geometrically this forms the hypotenuse of a right triangle, whereby the length can be solved with use of the Pythagorean Theorem, or a^2+b^2=c^2. Hence the associated (Vector Power)=sqrt((real axis length)^2 + (imaginary axis length)^2) formula. Finally for both the Reactive & Active Power, the measure of the vectors length or magnitude is simply from the origin (0,0) to the associated location along the real (0,y) or imaginary (x,0) axes.
The material written above, should assist in the understanding of the affects a rotation by versor k can have on the double frequency Power vector, or its source vectors E & I. While only generalities are used, they do serve to guide you to the right solution when doing calculations and measurements of a specific circuit arrangement.
Note: If any errors or unintelligible sections are found, send me a PM and I will correct them ASAP.
Garrett MLast edited by garrettm4; 02-02-2012, 10:44 PM.
Comment
-
Notes on the Crystal Set
Notes on the Crystal Set
When measuring the velocity difference between underground and our ground, the two seperate receivers can be brought into phase unison by the use of a test oscillator. Both receivers must have a primary tank circuit, here you can place your measurement equipment.
The detected AM output, audio frequency, signal is immune to receiver phase shift. This is a property of A.M. detection. Use the audio to measure propagation delay.
Wind all coils in the same direction, this is a must! Brass is a very good primary material, good depth of penetration at broadcast frequencies. Wires and clip lead interconnection are the "Road to Absolute Defeat." Coils and Condensers must be merged into each other in broad surfaces. Stacked metal plates on insulators makes good condensers. Transmitter air condensers are good, vacuum condensers are the best. A number of small condensers are better than one big one, less stray inductance. Stray inductance is fatal to success! For smaller units silver mica condensers are the only way, use no others. Paralell them on a low inductance bus.
Do not get sidetracked, experimental and auxilliary coils as well as patent diagrams will get you stuck in the sand forever. The finished Colorado unit dimensions are all in the notebook. It is all there, look at it, not the diversions, and remember everything you thought was Tesla is the exact opposite. It is there; one or two turn primary; a 20% height/width secondary; and a 100% height/width extra coil, all there, wire size, turns, all there, can you find it? It then can be scaled to any frequency and any KVAR capacity within wavelength conditions.
As your unit gets perfected the signal gets stronger and stronger, then diodes start burning out. We then are reaching the engineering objective, that is to light a number 327 pilot lamp. Not much power but too bright for Einstein.SUPPORT ERIC DOLLARD'S WORK AT EPD LABORATORIES, INC.
Purchase Eric Dollard's Books & Videos: Eric Dollard Books & Videos
Donate by Paypal: Donate to EPD Laboratories
Comment
-
Quadrapolar Resonance
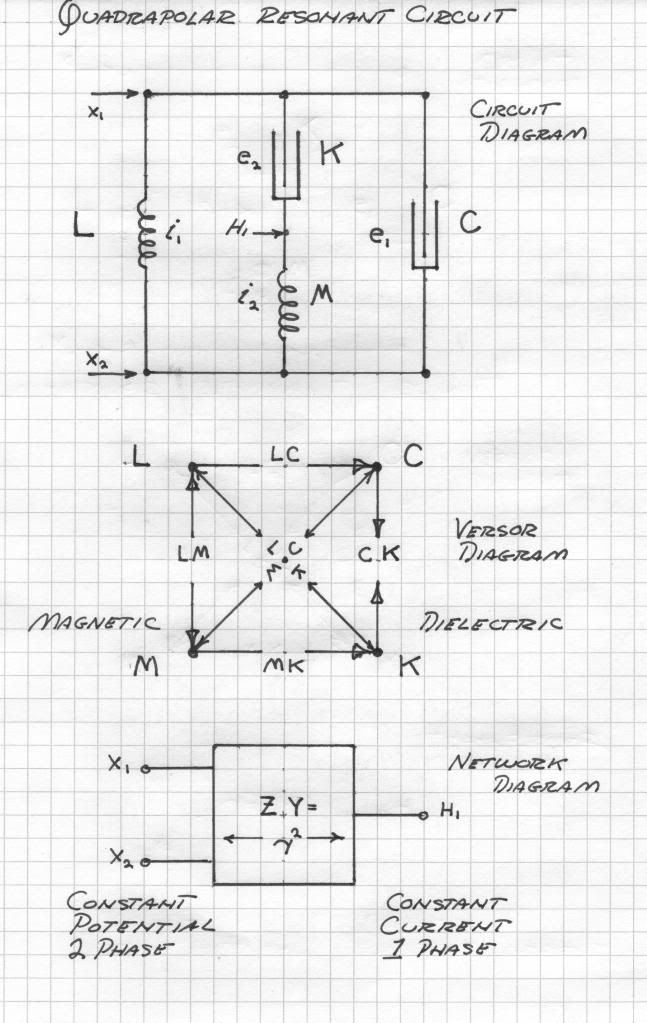

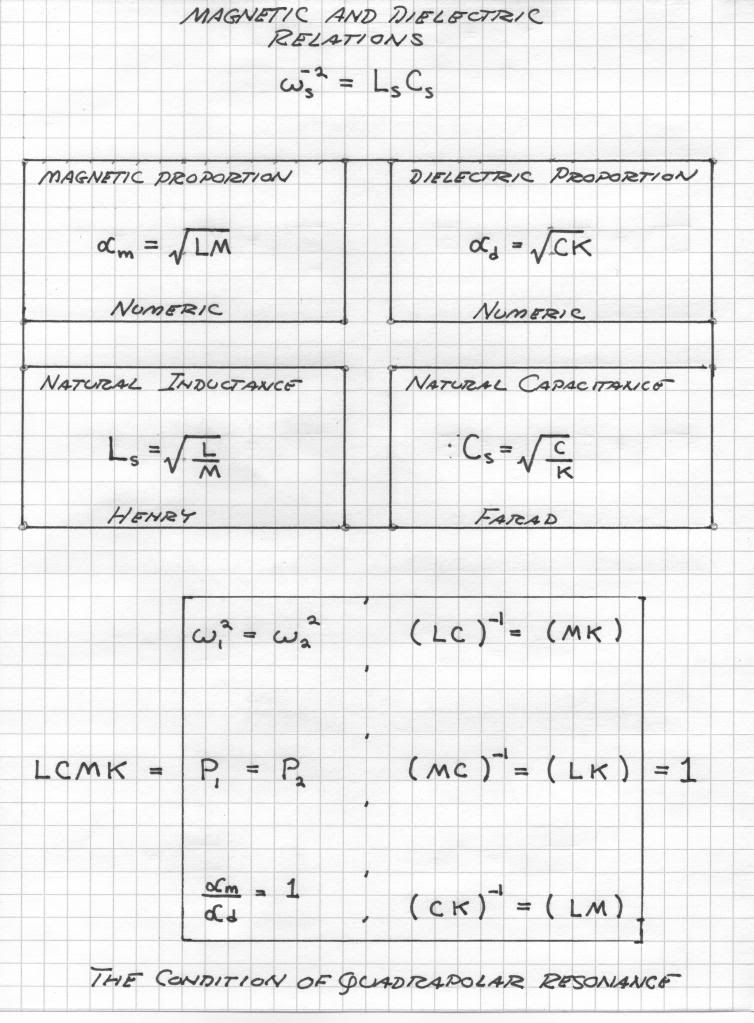 SUPPORT ERIC DOLLARD'S WORK AT EPD LABORATORIES, INC.
SUPPORT ERIC DOLLARD'S WORK AT EPD LABORATORIES, INC.
Purchase Eric Dollard's Books & Videos: Eric Dollard Books & Videos
Donate by Paypal: Donate to EPD Laboratories
Comment
-
Thank you! I've been trying to resolve the inductance, capacitance and resonance of a coil and it's dimensions to the wavelength. I have not till now read thru the springs notes. Tesla's notes are quite clear on the relations and how it's derived. First time I've seen the relationship explained.Originally posted by T-rex View PostNotes on the Crystal Set
When measuring the velocity difference between underground and our ground, the two seperate receivers can be brought into phase unison by the use of a test oscillator. Both receivers must have a primary tank circuit, here you can place your measurement equipment.
The detected AM output, audio frequency, signal is immune to receiver phase shift. This is a property of A.M. detection. Use the audio to measure propagation delay.
Wind all coils in the same direction, this is a must! Brass is a very good primary material, good depth of penetration at broadcast frequencies. Wires and clip lead interconnection are the "Road to Absolute Defeat." Coils and Condensers must be merged into each other in broad surfaces. Stacked metal plates on insulators makes good condensers. Transmitter air condensers are good, vacuum condensers are the best. A number of small condensers are better than one big one, less stray inductance. Stray inductance is fatal to success! For smaller units silver mica condensers are the only way, use no others. Paralell them on a low inductance bus.
Do not get sidetracked, experimental and auxilliary coils as well as patent diagrams will get you stuck in the sand forever. The finished Colorado unit dimensions are all in the notebook. It is all there, look at it, not the diversions, and remember everything you thought was Tesla is the exact opposite. It is there; one or two turn primary; a 20% height/width secondary; and a 100% height/width extra coil, all there, wire size, turns, all there, can you find it? It then can be scaled to any frequency and any KVAR capacity within wavelength conditions.
As your unit gets perfected the signal gets stronger and stronger, then diodes start burning out. We then are reaching the engineering objective, that is to light a number 327 pilot lamp. Not much power but too bright for Einstein.
Comment
Comment