Hi all,
I'm sure many of you know about moon-bouncing in amateur radio:
EME (communications) - Wikipedia, the free encyclopedia
In my Einstein article I added this:
Tuks DrippingPedia : Ruins 106 Years Einstein Relativity
Since the distance between the Earth and the Moon is on average 384,400 km, on average we would have a return path of over 750,000 km, which would take more than 2.5 seconds at the speed of light, which is the speed with which EM waves propagate. However, longitudinal waves could make the round-trip in just 1.6 seconds, a difference of 0.9 seconds!
I mean, explaining away a couple of nanoseconds in the CERN neutrino "anomaly", all right, they get away with that. But I would really love to see how they would explain away an early arrival of no less than 0.9 seconds...
So, if we were to make a longitudinal transmitter of considerable power (Wikipedia says you need over 100W for EM waves, but the higher the gain of your antenna's, the less power you need) and we would connect that to a computer (transmitting f.e. audible morse code or something), you could setup a system whereby any (radio amateur) could enter a message trough the internet to a server, connected to a transmitter which sends the signal to the moon. Now since any decent internet connection should be able to get the message from the amateurs computer to the transmitter computer well within half a second, any radio amateur that builds a receiver should be able to determine that the signal he himself entered on the computer is being received from the moon well before any EM wave could possibly travel back and forth between the earth and the moon.
That way, it could be experimentally proven that:
a) longitudinal waves exist,
b) they travel at much a faster speed than the speed of light.
This would mean that it could be experimentally proven that the current Maxwell equations are wrong and that therefore the Lorentz transform is rubbish after all and thus Einsteinan relativity can finally be put where it belongs: the trash can.
Since radio amateurs have been using moon-bouncing before, such an experiment using longitudinal waves may be possible without requiring large amounts of funding.
So, I went looking for some information on how to do this in practice, and it seems that all you need to be able to transmit and/or recieve longitudinal waves is spherical antenna:
Monstein, Wesley - Observation of scalar longitudinal electrodynamic waves(2002).pdf
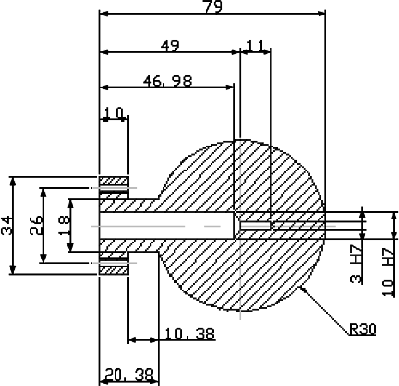
This is the sketch of the aluminium ball antennas from the pdf:
Now if you would mount such antenna's on a set of ordinary sattellite dishes, you could perhaps easily build longitudinal antenna with high gain, as some people do for the transmission of EM WiFi signals:
How-To: Build a WiFi biquad dish antenna -- Engadget
TREVOR MARSHALL - Biquad feed for primestar dish
It seems to me that this is not only doable, but also doable within a reasonable hobby-budget.
So, which radio amateur is going to get his name in the history books for performing not only the first longitudinal moon-bounce, but also delivering the final blow to Einstein's nonsense known as his relativity theory?
I'm sure many of you know about moon-bouncing in amateur radio:
EME (communications) - Wikipedia, the free encyclopedia
The use of the Moon as a passive communications satellite was proposed by Mr. W.J. Bray of the British General Post Office in 1940. It was calculated that with the available microwave transmission powers and low noise receivers, it would be possible to beam microwave signals up from Earth and reflect off the Moon. It was thought that at least one voice channel would be possible.
The "moon bounce" technique was developed by the United States Military in the years after World War II, with the first successful reception of echoes off the Moon being carried out at Fort Monmouth, New Jersey on January 10, 1946 by John H. DeWitt as part of Project Diana. The Communication Moon Relay project that followed led to more practical uses, including a teletype link between the naval base at Pearl Harbor, Hawaii and United States Navy headquarters in Washington, DC. In the days before communications satellites, a link free of the vagaries of ionospheric propagation was revolutionary.
Later, the technique was used by non-military commercial users, and the first amateur detection of signals from the Moon took place in 1953.
The "moon bounce" technique was developed by the United States Military in the years after World War II, with the first successful reception of echoes off the Moon being carried out at Fort Monmouth, New Jersey on January 10, 1946 by John H. DeWitt as part of Project Diana. The Communication Moon Relay project that followed led to more practical uses, including a teletype link between the naval base at Pearl Harbor, Hawaii and United States Navy headquarters in Washington, DC. In the days before communications satellites, a link free of the vagaries of ionospheric propagation was revolutionary.
Later, the technique was used by non-military commercial users, and the first amateur detection of signals from the Moon took place in 1953.
Tuks DrippingPedia : Ruins 106 Years Einstein Relativity
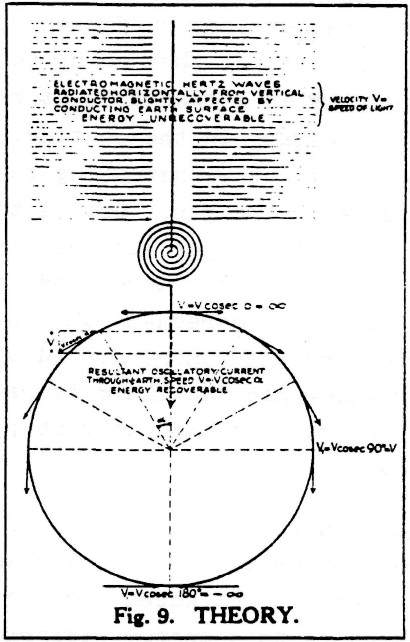
Given that the propagation speed of longitudinal electric waves (which according to the current theory cannot propagate trough a vacuum) is about 1.6 times the speed of light, it would be a very interesting experiment to see whether or not moon bouncing could be achieved practically with longitudinal electric waves. If Tesla is right, we would see an Earth-Moon-Earth roundtrip time of in the order of 1.6 seconds, while normal EM waves would take more than 2.5 seconds.
I mean, explaining away a couple of nanoseconds in the CERN neutrino "anomaly", all right, they get away with that. But I would really love to see how they would explain away an early arrival of no less than 0.9 seconds...

So, if we were to make a longitudinal transmitter of considerable power (Wikipedia says you need over 100W for EM waves, but the higher the gain of your antenna's, the less power you need) and we would connect that to a computer (transmitting f.e. audible morse code or something), you could setup a system whereby any (radio amateur) could enter a message trough the internet to a server, connected to a transmitter which sends the signal to the moon. Now since any decent internet connection should be able to get the message from the amateurs computer to the transmitter computer well within half a second, any radio amateur that builds a receiver should be able to determine that the signal he himself entered on the computer is being received from the moon well before any EM wave could possibly travel back and forth between the earth and the moon.
That way, it could be experimentally proven that:
a) longitudinal waves exist,
b) they travel at much a faster speed than the speed of light.
This would mean that it could be experimentally proven that the current Maxwell equations are wrong and that therefore the Lorentz transform is rubbish after all and thus Einsteinan relativity can finally be put where it belongs: the trash can.
Since radio amateurs have been using moon-bouncing before, such an experiment using longitudinal waves may be possible without requiring large amounts of funding.
So, I went looking for some information on how to do this in practice, and it seems that all you need to be able to transmit and/or recieve longitudinal waves is spherical antenna:
Monstein, Wesley - Observation of scalar longitudinal electrodynamic waves(2002).pdf
Mathematically a spherically symmetric source can generate only scalar waves; so the ball antenna can only generate a Φ-wave, and, thus, only a longitudinal electrodynamic E -wave. The spherically symmetric current density J within the ball, that gives rise to the pulsating surface charge source, is divergenceless, ∇ · J = 0; so ∇ · A = 0 and ∇× A = 0; and no transverse wave can arise. The ball antenna as a receiver detects the net charge induced by the component of the incident E field normal to the front surface; so only longitudinal E-waves can be detected.

Now if you would mount such antenna's on a set of ordinary sattellite dishes, you could perhaps easily build longitudinal antenna with high gain, as some people do for the transmission of EM WiFi signals:
How-To: Build a WiFi biquad dish antenna -- Engadget
TREVOR MARSHALL - Biquad feed for primestar dish
It seems to me that this is not only doable, but also doable within a reasonable hobby-budget.
So, which radio amateur is going to get his name in the history books for performing not only the first longitudinal moon-bounce, but also delivering the final blow to Einstein's nonsense known as his relativity theory?

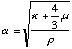
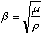

Comment